Fidelio, Vol. VIII,
No 1-2,  Spring/Summer 2004

Knowing the True Geometry,
A Dialogue in Two Parts

Q: I read last week’s pedagogical discussion, and I believe there is an error, or at least a contradiction, in it.

A: That is certainly possible, but it is also likely that the error, or contradiction, arises from a lack of comprehension of these matters. Thinking about these matters teases the most profound concepts from our spirit. Let’s have at it, and find out from whence this error comes, or at least provoke enough thought to get us stirred up about it.

Q: Well, first of all, I don’t quite understand the question of the parallel postulate. Is the question whether parallel lines exist?

A: Not really. As a matter of historical literacy, you should be familiar with the definitions and postulates of Euclid’s Elements. The Elements begins with 23 definitions, 5 postulates, and 8 common notions, which lay the foundation from which the subsequent geometrical theorems and constructions are demonstrated. The definitions describe the objects of Euclidean geometry, such as points, lines, circles, straight lines, right angles, and parallel lines. The postulates describe assumptions about the nature of space which, for Euclid, were self-evident and not necessary to demonstrate, nor susceptible of it.

The postulates are:

1. To draw a straight line from any point to any point.

2. To produce a finite straight line continuously in a straight line.

3. To describe a circle with any center and diameter.

4. All right angles are equal to one another.

5. If a straight line falling on two straight lines make the interior angles on the same side less than two right angles, the two straight lines, if produced indefinitely, meet on that side on which are the angles less than two right angles. [See Figure 1]

Figure 1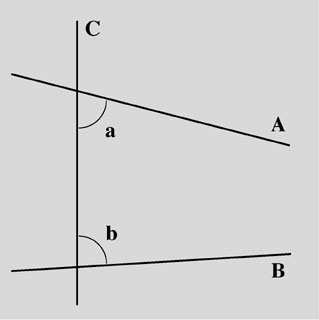Euclid’s “parallel postulate”: If ∠a + ∠b < 180°, then lines A and B converge.

The first three postulates are constructions that describe the assumption that space is infinite and continuous. The fourth postulate describes the assumption that figures in space don’t change their shape with changes in position. It is the fifth, convoluted, negatively stated postulate that drew the attention of Kästner, Gauss, and Riemann, as it had for so many others over the preceding 2,000 years. The troubling question is, is it self-evident that the two straight lines will converge when extended indefinitely? Or, stated inversely, if the two straight lines make two interior right angles with the third line, that they will never converge—that is, they will be what Euclid defines as parallel.

Figure 2The further the point of convergence, the the greater the interior angles.

Q: It certainly seems that two straight lines, so constructed, would converge, no matter how far they were extended, until the interior angles formed two right angles. If I make a drawing of two straight lines that converge, then I cut those lines with another straight line, it will form a triangle [See Figure 2]. It is clear from the drawing, that the two interior angles are less than two right angles. If I now make these interior angles larger, the two straight lines still converge, except at a point further from the third line. If I had a big enough piece of paper, I could make the interior angles as close to right angles as I wanted, and the drawing would show that these lines still converged. From this it seems self-evident, that when these two interior angles both become right angles, the two lines will not converge, but will become parallel instead.

A: That is exactly the argument Gauss had with H.C. Schumacher back in 1831. You are assuming as self-evidently true, that the infinite is merely the simple extension of the finite. Embedded in the idea that underlies your drawing, are the assumptions about space described in the first four of Euclid’s postulates, that is, that space is continuous, infinite, and homogeneous. In his letter to Schumacher, Gauss proposed that he consider what the same drawing would look like, if space were anti-Euclidean. In such a case, the triangles in your drawing would look quite different. Gauss depicted these triangles as formed by negatively curved arcs, similar to hypocycloids [See Figure 3].

Figure 3Anti-Euclidean, negatively curved triangle.

As these negatively curved triangles grew, the arcs would curve more and more inwardly towards the center of the triangle. But, an infinite triangle depicted in this way, would no longer appear as a triangle; instead, it would appear as three divergent lines intersecting in one point. This is a completely different figure from the finite one. If space is anti-Euclidean, than the infinite can not be depicted as a simple extension of the finite.

From the standpoint of Euclidean geometry, this discontinuity between the finite and the infinite would seem contradictory. In his 1831 correspondence, Gauss used this pedagogical example to criticize Schumacher for thinking of the infinite as a completed simple extension of the finite. Instead, Gauss says the infinite should be thought of as a boundary.

(Incidentally, Georg Cantor later criticized Gauss for rejecting the notion of a completed infinite; but, although Gauss did not have Cantor’s concept of the transfinite, Cantor must not have taken into consideration Gauss’s complete thoughts on this question.)

Gauss wrote Schumacher, “In this sense, anti-Euclidean geometry contains in it nothing contradictory, although those many results that at first must seem paradoxical, would be a contradiction owing only to a self-deception, brought forth by a prior habit of taking Euclidean geometry as rigorously true.” And he ends his letter: “There is nothing contradictory in this, as long as finite man doesn’t presume to want to regard something infinite, as given and capable of being comprehended by his habitual way of viewing things.”

Q: That brings me to the point that I originally thought was an error, or contradiction, last week. If Gauss says that paradoxes such as these arise from the habit of taking Euclidean geometry as rigorously true, how can we determine what the true geometry is? On the one hand, Kästner says, “[T]he basis of truth and certainty is not in the metaphor of the subject, but in the intelligibility, the conceptions of reason in which those metaphors lie. That, I would think, would be obvious from those geometrical theorems that are capable of being proven. One never concludes from the form, but from it, one thinks of the reason of the form.” From this standpoint, it seems that one could determine the true geometry purely from the conceptions of reason that underlie it. Yet, the pedagogical went on to say, using the examples of Kepler, Gauss, and Riemann, that the only way to determine truth is in the domain of physics. Isn’t this contradictory?

A: Now we’re at the problem. Look again at Kepler’s revolution over Ptolemy, Brahe, and Copernicus. Kepler rejected those three models, not merely because their results deviated from physical observation, but because they were merely models, and could not, and did not intend to, distinguish the truth underlying the physical observations [See Figure 4(a)-(c)].

Figure 4(a) Ptolemaic system:Earth-centered, constant circular action.

Figure 4(b) 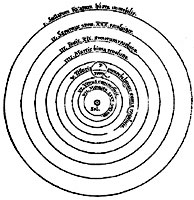Copernican system: sun-centered, constant circular action.

Figure 4(c) 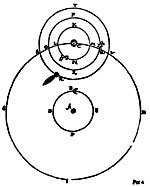Brahe’s system: mixed Earth-and sun-centered, constant circular action.

All three derived the physically observed non-uniform motion of the planets, from mathematics constructed from constant circular action; whereas Kepler constructed a mathematics of non-constant curvature, from the physically observed non-uniform motion [See Figure 5].

Figure 5Keplerian system: Non-constant motion on an elliptical orbit. The ratios of swept-out areas. In equal time intervals, therefore, the areas of the curvilinear sectors swept out by the planet, will b e equal—even though the curvilinear distances traversed on the orbit are constantly changing. In the region about perihelion, nearest the sun, the planet moves fastest, covering the gratest orbital distance; whereas, at aphelion, farthest from the sun, it moves most slowly, covering the least distance.

The validity of Kepler’s hypothesis was then demonstrated, by the unique experiment of the physical measurement of the planetary orbits, especially with Gauss’ later determination of the orbit of the asteroid Ceres.

So too is the case, as Gauss points out, with Euclidean geometry. As Gauss shows, you cannot attribute to physical space the characteristics of Euclidean geometry, just because we are habituated to viewing things that way. But, how then are we to determine the characteristics of geometry? It could not be simply a matter of replacing the parallel postulate, with some other postulate, because that would lead us into the same predicament as that of Ptolemy, Brahe, and Copernicus. We would still be unable to determine which geometry is the true one, unless we constructed that geometry out of the paradoxes of physical measurement, as Kepler did. For the same reasons that Kepler rejected the models of Ptolemy, Brahe, and Copernicus, Gauss became convinced when he was 15 years old, that Euclidean geometry was not the true one. It was not the true one, because it could not be proven true, no matter how accustomed we were to its results. To determine the characteristics of the true geometry, Gauss followed the method of Kepler: He constructed physical measurements in the domain of geodesy, electrodynamics, geomagnitism and astronomy, which were continued by Riemann, Weber, Fresnel, Ampere, and others.

And this takes us into another higher domain, the domain of physical economy as developed by Lyndon LaRouche, a domain one can come to know best by studying LaRouche’s works directly.

* * *

Q: I’ve been reading the last pedagogical discussions on anti-Euclidean geometry, and I still get confused when I try to think of different geometries. The only thing that comes to mind is the difference between a sphere and plane, which difference I think of in terms of the difference between the characteristics of triangles on a plane and on a sphere. Is this what is meant by anti-Euclidean geometry?

A: No. The problem isn’t with the images you describe, but with the way in which you think of those images. You have to direct your mind toward the concepts underlying the way you think of geometry. While one may proceed, at first, to the concepts through images, the concepts are not found in the images.

Think of how Abraham Gotthelf Kästner concludes his essay, “On the Mathematical Concept of Space”:

“[T]he basis of truth and knowledge does not lie in the sense notion [or image-N-BD] of the subject, but in the intelligible concept: in the concepts of reason concerning the cause of the sense image.

“This, I would imagine, should be clear for the teachings of geometry, to everyone who can grasp its proofs. We never draw conclusions from the [visual-N-BD] images, but only from that which our mind thinks about them ... .”

Q: So, what are the concepts that underlie the images of Euclidean and anti-Euclidean geometries, and how can we distinguish which geometry is the true one?

A: We can arrive at these concepts pedagogically by recreating Gauss’s discoveries, and the earlier investigations of his teacher Kästner. The first step is to force your mind to recognize the underlying assumptions of Euclidean geometry. But, first and foremost, you have to recognize that those assumptions exist, and then grasp the way in which they arise, and how they remain hidden from your conscious thought. This is what Kästner and Gauss tried so hard to get their colleagues to understand.

First, recall to mind the 1831 correspondence between Schumacher and Gauss, on the subject of parallel lines. In May 1831, Schumacher proposed to Gauss a proof of the truth of Euclid’s parallel postulate, by demonstrating that the sum of the angles of a triangle is always 180 degrees. While Schumacher’s proof was formally valid, Gauss pointed out a devastating error in his friend’s thinking. Underlying Schumacher’s demonstration was the assumption that space was infinitely extended and had zero curvature. In such a space, straight lines would behave as Schumacher indicated. But, if space were bounded and curved, straight lines would behave differently. According to Gauss, Schumacher’s assumption arose “from an early habituation to thinking of Euclidean geometry as rigorously true.”

Figure 6 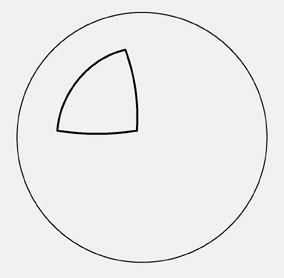The sides of a triangle drawn on a sphere are curved.

Q: That is why I thought of the image of comparing triangles on a sphere with triangles on a plane, since the sphere is bounded and curved, while the plane is infinitely extended and flat. Thus, the triangles on the sphere have curved sides, while the triangles on the plane have straight sides [See Figure 6].

A: You are falling into the trap Kästner warns about. You are looking for certainty and truth in the images, not in the concepts. Why do you say the triangles on the sphere have curved sides?

Do you remember a pedagogical discussion written by Jonathan Tennenbaum back in 1998, in which he posed the experiment of drawing an equator on a sphere and two great circles that intersect that equator? When you look at those great circles from the center of the sphere, they appear to be straight lines. In fact, the image you see appears to be two parallel straight lines, intersecting another straight line at 90 degrees [See Figure 7].

Figure 7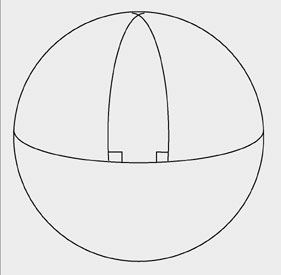Great circles on a sphere. Seen from the sphere’s center, the lines appear to be parallel at the equator, but converge at the pole.

Q: I do remember that experiment, and I remember being startled by the result.

A: Then you surely remember that when you looked straight ahead to the equator, the lines looked parallel, but when you turned your eye toward the pole, the lines began to converge.

Q: That is what I recall being so surprising.

A: Well, Gauss posed a similar paradox to Schumacher. If space had a negative curvature, then the sum of the angles of a triangle would be less than 180 degrees. The sides of such triangles depicted on a flat piece of paper would look like hypocycloids, although, as discussed last week, this is just a depiction. But, if space were negatively curved, the sides of the triangles, whose angles added up to less than 180 degrees, would nevertheless be “straight” lines.

Q: I’m having trouble visualizing such a concept.

A: For exactly the reason stated by Gauss above. Visualizing this idea seems to contradict your assumptions, because when you think of triangles whose angles add up to less than 180 degrees, you think of their depiction in Euclidean space. As such, you can only think of such triangles as having curved sides. But, if space were not Euclidean, this would not be a contradiction. As Gauss told Schumacher: “Anti-Euclidean geometry contains nothing contradictory, although some people at first will consider many of its results paradoxical—the which, however, to consider as contradictory, would be a self-deception, arising from an early habituation to thinking of Euclidean geometry as rigorously true.”

Q: This will take some thinking.

A: That’s the idea. Now, refresh your recollection of the Euclid’s parallel postulate by drawing two straight lines on a piece of paper and label them A and B [Figure 1]. Then draw a third line C, that cuts the other two. The parallel postulate says that lines A and B will intersect if the angles they make with C are less than 180 degrees. Conversely, if the angles are greater than 180 degrees they will diverge. If the angles are equal to 180 degrees they will never meet.

Q: That I remember.

A: Now think of how the two images we just constructed pose a contradiction with what you just imagined. In the one by Tennenbaum, the straight lines A and B would be the great circles that intersect the equator C at 180 degrees. According to the parallel postulate, they should never meet, yet, in fact, they converge at the pole. In the image posed by Gauss, the straight lines A and B intersect C at less than 180 degrees [Figure 3]. According to the parallel postulate, they should intersect, but if space were negatively curved, these lines would converge but never intersect.

Q: How can it be that we have three sets of three lines, all configured in the same way, and yet get three entirely different results?

A: Now you’re beginning to see the paradox that Gauss and Kästner posed, to get people to recognize the underlying assumptions they had made about the nature of space. In a certain sense, this is an inversion of the problem Kepler posed with respect to the geometries of Ptolemy, Brahem and Copernicus. In that case, three entirely different configurations yielded the equivalent result. In the paradox posed here, three equivalent configurations yield entirely different results. Nevertheless the same principle is demonstrated: the primary role of cognition.

Now go back and think about why you thought the parallel postulate of Euclid was true in the first place.

Q: Because when I drew the picture, the two lines always intersected, as long as I extended them far enough.

A: In other words, you thought it was true, because it always worked.

Q: Yes, and there was nothing to indicate it would be otherwise.

A: And you assumed that if it worked in a small region of space, it would continue to work in a large, or even infinite region of space.

Q: Well, why not? There is nothing to indicate it shouldn’t. I even tried it with a very large piece of paper, and the lines still intersected.

A: That’s exactly the type of thinking that’s leading the world to disaster. Think of the poor Baby Boomer who thinks his mutual funds will reach a certain amount so he can retire at a certain age, before his mortal body deteriorates to the point where he can no longer experience sensual pleasure. He assumes the two lines (his mutual funds and his age), will intersect at some point (his retirement), simply because they appear to converge prior to another point (his physicaly decay). But this image is based on an assumption about the nature of the space in which these points and lines lie. If the nature of space is like the one Gauss proposes, the two lines will never meet, and the Baby Boomer will never have the retirement he so earnestly desires. And to make things even more complicated, what if the nature of space changes, as the lines are extended? Such an image, as Riemann showed, is closer to reality. But for now, let’s stick to the simpler case, which is sufficient to demonstrate the point.

Q: How, then, can I know the underlying nature of space, if no matter what the curvature, the straight lines will appear “straight?” How can I know if these lines will converge, diverge, or be parallel?

Carl F. Gauss wrote of the seeming paradoxes of anti-Euclidean geometry: “There is nothing contradictory on this, as long as finite man doesn’t mistake something infinite, as something given, and thus capable of being comprehended by his habitual way of viewing things.”

A: You must reverse your thinking entirely. Previously, you deluded yourself into thinking that the infinite would be the same as the finite, only more of it. You assumed the parallel postulate to be true, because it appears to work in finite regions of space, and you assumed it would continue to work for the infinite. Now, reverse your thinking. Let the infinite determine the finite. If the infinite is bounded, in the sense of Cantor’s transfinite, or, Gauss’s anti-Euclidean geometry, then the finite relations are different from what they would be, if you assume the infinite was a simple extension of the finite. In fact, your assumptions about finite space are derived from your conception of the infinite; anything else is self-deception.

As Gauss told Schumacher in 1831 with respect to this very paradox, “There is nothing contradictory in this, as long as finite man doesn’t mistake something infinite, as something given, and thus capable of being comprehended by his habitual way of viewing things.”

In future issues we will investigate this further.

A translation of excerpts from Abraham Kästner’s “On the Conceptions that Underlie Space” appears in this issue.

top of page